The Jedell Report: 2019

By David William Jedell August 24, 2022

Einstein's Field Equations

-----------Warp Drive Progress----------- https://apple.news/AOG5_oNtCNai8_oi2JVJQCg ---------------------------------------------------------------------------------- -----Gravitation as a Casimir Effect https://www.diva-portal.org/smash/get/diva2:214280/FULLTEXT01.pdf ---------------------------------------- Gravitational Casimir Effect - Gravitons-- https://arxiv.org/pdf/1502.07429.pdf -----------------------------------------------Stanford General Relativity-----------Leonard Susskind Lecture Series---------https://m.youtube.com/watch?v=JRZgW1YjCKk ----------------------------------------- -----The Field Equation and Curvature------ Most of us have heard of Einstein's equations which describe the universe around us, yet only some of us understand what the equations are actually saying.

The Einstein field equations (EFE) may be written in the form:

R_{\mu \nu }-{\tfrac {1}{2}}Rg_{\mu \nu }+\Lambda g_{\mu \nu }={\frac {8\pi G}{c^{4}}}T_{\mu \nu }

where

R μν

is the Ricci curvature tensor,

R

is the scalar curvature,

g μν

is the metric tensor,

Λ

is the cosmological constant,

G

is Newton's gravitational constant,

c

is the speed of light in vacuum, and

T μν

is the stress–energy tensor.

Einstein's equations can be loosely summarized as the main relation between matter and the geometry of spacetime. I will try to give a qualitative description what every term in the equation signifies. I will, however, have to warn potential readers that this will not be a short answer.

Despite the fact that it's over a century old, Einstein's theory of general relativity is our current understanding of how gravity operates. In this view, space and time are merged together into a unified framework known as (no surprises here) space-time. This space-time isn't just a fixed stage but bends and flexes in response to the presence of matter and energy. That bending, warping and flexing of space-time then goes on to tell matter how to move. In general relativity, everything from bits of light to speeding bullets to blasting spaceships want to travel in straight lines. But the space-time around them is warped, forcing them all to follow curved trajectories — like trying to cross a mountain pass in a straight line, but following the peaks and valleys of the topography.

Matter

On the right hand side of the equation, the most important thing is the appearance of the energy-momentum tensor

T_{μ ν}

. It encodes exactly how the matter---understood in a broad sense, i.e. any energy (or mass or momentum or pressure) carrying medium---is distributed in the universe. For understanding how to interpret the subscript indices of the

T

, see my explanation of the metric tensor below.
It is multiplied by some fundamental constants of nature (the factor

\frac{8 π G}{c^{4}})

but this isn't of any crucial importance: One can view them as book-keeping tools that keep track of the units of the quantities that are related by the equation. In fact, professional physicists typically take the liberty to redefine our units of measurements in order to simplify the look of our expressions by getting rid of pesky constants such as this. One particular option would be to choose "reduced Planck units", in which

8 π G = 1

and

c = 1

, so that the factor becomes

1

Differential geometry

On the left hand side of Einstein's equations, we find a few different terms, which together describe the geometry of spacetime. General relativity is a theory which uses the mathematical framework known as (semi-)Riemannian geometry. In this branch of mathematics, one studies spaces which are in a certain sense smooth, and that are equipped with a metric. Let us first try to understand what these two things mean.
The smoothness property can be illustrated by the intuitive (and historically important!) example of a smooth (two-dimensional) surface in ordinary three-dimensional space. Imagine, for instance, the surface of an idealized football, i.e. a 2-sphere. Now, if one focuses ones attention to a very small patch of the surface (hold the ball up to your own face), it seems like the ball is pretty much flat. However, it is obviously not globally flat. Without regards for mathematical rigor, we can say that spaces that have this property of appearing locally flat are smooth in some sense. Mathematically, one calls them manifolds. Of course, a globally flat surface such as an infinite sheet of paper is the simplest example of such a space.
In Riemannian geometry (and differential geometry more generally) one studies such smooth spaces (manifolds) of arbitrary dimension. One important thing to realize is that they can be studied without imagining them to be embedded in a higher-dimensional space, i.e. without the visualization we were able to use with the football, or any other reference to what may or may not be "outside" the space itself. One says that one can study them, and their geometry, intrinsically.

The metric

When it comes to intrinsically studying the geometry of manifolds, the main object of study is the metric (tensor). Physicists typically denote it by

g_{μ ν}

. In some sense, it endows us with a notion of distance on the manifold. Consider a two-dimensional manifold with metric, and put a "coordinate grid" on it, i.e. assign to each point a set of two numbers,

(x, y)

. Then, the metric can be viewed as a

2 \times 2

matrix with

2^{2} = 4

entries. These entries are labeled by the subscripts

μ, ν

, which can each be picked to equal

x

y

. The metric can then be understood as simply an array of numbers:

(g x x g y x g x y g y y)

We should also say that the metric is defined such that

g_{μ ν} = g_{ν μ}

, i.e. it is symmetric with respect to its indices. This implies that, in our example,

g_{x y} = g_{y x}

. Now, consider two points that are nearby, such that the difference in coordinates between the two is

(d x, d y) .

We can denote this in shorthand notation as

d l^{μ}

where

μ

is either

x

y,

and

d l^{x} = d x

and

d l^{y} = d y .

Then we define the square of the distance between the two points, called

d s,

d s 2 = g x x d x 2 + g y y d y 2 + 2 g x y d x d y = \sum μ, ν \in {x, y} g μ ν d l μ d l ν

To get some idea of how this works in practice, let's look at an infinite two-dimensional flat space (i.e. the above-mentioned sheet of paper), with two "standard" plane coordinates

x, y

defined on it by a square grid. Then, we all know from Pythagoras' theorem that

d s 2 = d x 2 + d y 2 = \sum μ, ν \in {x, y} g μ ν d l μ d l ν

This shows that, in this case, the natural metric on flat two-dimensional space is given by

g μ ν = (g x x g x y g x y g y y) = (1001)

Now that we known how to "measure" distances between nearby points, we can use a typical technique from basic physics and integrate small segments to obtain the distance between points that are further removed:

L = \int d s = \int \sum μ, ν \in {x, y} g μ ν d l μ d l ν - - - - - - - - - - - - - \sqrt

The generalization to higher dimensions is straightforward.

Curvature tensors

As I tried to argue in the above, the metric tensor defines the geometry of our manifold (or spacetime, in the physical case). In particular, we should be able to extract all the relevant information about the curvature of the manifold from it. This is done by constructing the Riemann (curvature) tensor

R_{ν ρ σ}^{μ}

, which is a very complicated object that may, in analogy with the array visualization of the metric, be regarded as a four-dimensional array, with each index being able to take on

N

values if there are

N

coordinates

{x^{1}, \dots x^{N}}

on the manifold (i.e. if we're dealing with an

N

-dimensional space). It is defined purely in terms of the metric in a complicated way that is not all too important for now. This tensor holds pretty much all the information about the curvature of the manifold---and much more than us physicists are typically interested in. However, sometimes it is useful to take a good look at the Riemann tensor if one really wants to know what's going on. For instance, an everywhere vanishing Riemann tensor (

R_{ν ρ σ}^{μ} = 0

) guarantees that the spacetime is flat. One famous case where such a thing is useful is in the Schwarzschild metric describing a black hole, which seems to be singular at the Schwarzschild radius

r = r_{s} \neq 0

. Upon inspection of the Riemann tensor, it becomes apparent that the curvature is actually finite here, so one is dealing with a coordinate singularity rather than a "real" gravitational singularity.
By taking certain "parts of" the Riemann tensor, we can discard some of the information it contains in return for having to only deal with a simpler object, the Ricci tensor:

R ν σ : = \sum μ \in {x 1, \dots x N} R μ ν μ σ

This is one of the tensors that appears in the Einstein field equations. the second term of the equations features the Ricci scalar

R

, which is defined by once again contracting (a fancy word for "summing over all possible index values of some indices") the Ricci tensor, this time with the inverse metric

g^{μ ν}

which can be constructed from the usual metric by the equation

\sum ν \in {x 1, \dots, x N} g μ ν g ν ρ = 1 if μ = ρ and 0 otherwise

As promised, the Ricci scalar is the contraction of the Ricci tensor and inverse metric:

R : = \sum μ, ν \in {x 1, \dots x N} g μ ν R μ ν

Of course, the Ricci scalar once again contains less information than the Ricci tensor, but it's even easier to handle. Simply multiplying it by

g_{μ ν}

once again results in a two-dimensional array, just like

R_{μ ν}

and

T_{μ ν}

are. The particular combination of curvature tensors that appears in the Einstein field equations is known as the Einstein tensor

G μ ν : = R μ ν - 1 2 R g μ ν

The cosmological constant

There is one term that we have left out so far: The cosmological constant term

Λ g_{μ ν}

. As the name suggests,

Λ

is simply a constant which multiplies the metric. This term is sometimes put on the other side of the equation, as

Λ

can be seen as some kind of "energy content" of the universe, which may be more appropriately grouped with the rest of the matter that is codified by

T_{μ ν}

.

Einstein's equation relates the matter content (right side of the equation) to the geometry (the left side) of the system. It can be summed up with "mass creates geometry, and geometry acts like mass".
For more detail, let's consider what a tensor is. A two-index tensor (which is what we have in Einstein's equation), can be thought of as a map which takes one vector into another vector. For example, the stress-energy tensor takes a position vector and returns a momentum vector, mathematically

p_{ν} = T_{ν μ} x^{μ}

The interpretation is that the right side of Einstein's equation tells us the momentum which is passing through a surface defined by the position vector.
The left side can be interpreted in this manner as well. The Ricci curvature

R_{μ ν}

takes a position vector and returns a vector telling us how much the curvature is changing through the surface defined by

\vec{x}

. The second and third terms, both having factors of the metric

g_{μ ν}

, tell us how much distance measurements are changed when traveling along the vector. There are two contributions to this change in distance - the scalar curvature

R

and the

Λ

. If

R_{μ ν}

is "curvature in a single direction", than

R

is the "total curvature".

Λ

is a constant which tells us how much innate energy empty space has, making all distances get larger for

Λ > 0

.
So, reading the equation right to left, "Einstein's equation tells us that momentum (moving mass) causes both curvature and a change in how distances are measured." Reading left to right, "Einstein's equation tells us that curvature and changing distance acts just like moving mass."

Despite its intricacy, relativity remains the most accepted way to account for the physical phenomena we know about. Yet scientists know that their models are incomplete because relativity is still not fully reconciled with quantum mechanics. which explains the properties of subatomic particles with extreme precision but does not incorporate the force of gravity.

Alcubierre “Warp” Drive

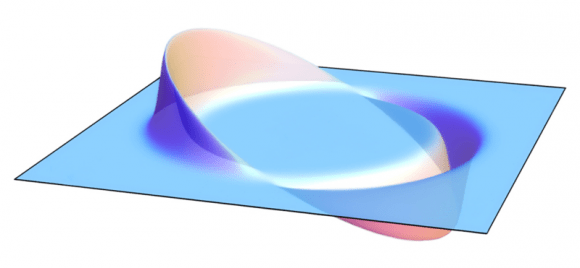
To put it simply, this method of space travel involves stretching the fabric of space-time in a wave which would (in theory) cause the space ahead of an object to contract while the space behind it would expand. An object inside this wave (i.e. a spaceship) would then be able to ride this region, known as a “warp bubble” of flat space.
This is what is known as the “Alcubierre Metric”. Interpreted in the context of General Relativity, the metric allows a warp bubble to appear in a previously flat region of spacetime and move away, effectively at speeds that exceed the speed of light. The interior of the bubble is the inertial reference frame for any object inhabiting it.
In 1994, a Mexican physicist by the name of Miguel Alcubierre came along with proposed method for stretching the fabric of space-time in a way which would, in theory, allow Faster Than Light travel to take place.

Image result for Einstein Field Equation Warp

Image result for Einstein Field Equation Warp

Since the ship is not moving within this bubble, but is being carried along as the region itself moves, conventional relativistic effects such as time dilation would not apply. Hence, the rules of space-time and the laws of relativity would not be violated in the conventional sense.

Physicists may have just manipulated 'pure nothingness'

quantum fluctuations

How did they do this? Their method essentially involves firing a super short laser pulse lasting only a few femtoseconds (which, if you're counting, is measured on the level of millionths of a billionths of a second) into a "squeezed" vacuum. As the light fires through this vacuum, subtle changes in the polarization of the light can be analyzed to reveal a map, of sorts, of the quantum nothingness.

Furthermore, according the Stephen Hawking, the principle of equivalence, which says that gravity couples to the energy-momentum tensor of matter, and the quantum-mechanical requirement that energy should be positive imply that gravity is always attractive.

Faster Than Light Galaxies
All the galaxies in the Universe beyond a certain distance appear to recede from us at speeds faster than light. Even if we emitted a photon today, at the speed of light, it will never reach any galaxies beyond that specific distance. It means any events that occur today in those galaxies will not ever be observable by us. However, it's not because the galaxies themselves move faster than light, but rather because the fabric of space itself is expanding.

References

What is the Alcubierre "warp" drive?
https://phys.org/news/2017-01-alcubierre-warp.html

Layman's Understanding of Einstein's Field Equations
https://physics.stackexchange.com/questions/179082/laymans-explanation-and-understanding-of-einsteins-field-equations

What is the Alcubierre “Warp” Drive?
https://www.universetoday.com/89074/what-is-the-alcubierre-warp-drive/

S. W. Hawking: Breakdown of predictability in gravitational collapse
https://journals.aps.org/prd/abstract/10.1103/PhysRevD.14.2460

Unclassified Defense Intelligence Reference Document, Advanced Space Propulsion Based on Vacuum (Spacetime Metric) Engineering (March 29, 2010)

https://documents.theblackvault.com/documents/Advanced_Space_Propulsion_1525479713208_41686961_ver1.0.pdf

Dr. Harold “Sonny” White, NASA Johnson Space Center, Warp Field Mechanics 101 (September 2, 2011)

https://ntrs.nasa.gov/archive/nasa/casi.ntrs.nasa.gov/20110015936.pdf

Physicists may have just manipulated 'pure nothingness'
https://www.mnn.com/green-tech/research-innovations/stories/physicists-may-have-just-manipulated-pure-nothingness

Bergmann, Peter Gabriel; Einstein, Albert, Theory of Relativity, Dover Publications, Inc., (1976)

Mann, Adam, What is Space-Time, Live Science (Dec. 19, 2019)
https://www.livescience.com/space-time.html

Aron, Jacob, Quantum weirdness proved real in first loophole-free experiment, New Scientist (2015)
https://www.newscientist.com/article/dn28112-quantum-weirdness-proved-real-in-first-loophole-free-experiment/

Hensen, Bas; Kalb, Norbert; Blok, et. al, Loophole-free Bell test using electron spins in diamond, Second experiment and additional analysis, Delft University of Technology (2016)
http://pure.tudelft.nl/ws/files/11820176/srep30289.pdf

Siegel, Ethan, This Is How Distant Galaxies Recede Away From Us At Faster-Than-Light Speeds, Forbes (2019)
https://www.forbes.com/sites/startswithabang/2019/11/07/this-is-how-distant-galaxies-recede-away-from-us-at-faster-than-light-speeds/#5c080cc872a2

Sutter, Paul, The Universe Remembers Gravitational Waves — And We Can Find Them,
Space.com (12-6-2019)
https://www.space.com/gravitational-waves-memory-space-time.html

Copyright © 2022 David William Jedell
Email: d.w.jedell@gmail.com

The Jedell Report

Wednesday, September 25, 2019

Einstein's Field Equation Explains Faster than Light Warp Drive

Matter

Differential geometry

The metric

Curvature tensors

The cosmological constant

Time

Report Abuse